Understanding the formula for futures price is essential for making smart investment decisions and managing risks. Knowing how futures prices are calculated, you can determine if a futures contract is priced fairly, helping you spot potential profit opportunities. It also allows you to anticipate market movements and hedge against price fluctuations in the underlying asset. So without wasting time, have a look.
What are Future Contracts?
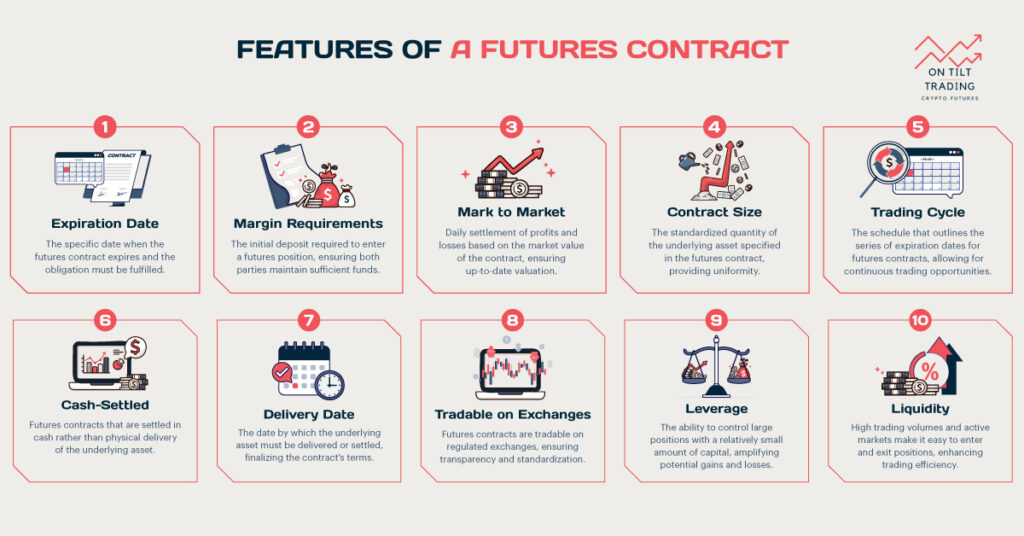
Futures contracts are standardized agreements to buy or sell a specific asset at a predetermined price on a specified future date. These contracts are traded on futures exchanges and cover a variety of assets, including commodities, currencies, and financial instruments.

Futures markets can be highly volatile, with rapid price changes that can result in substantial losses.
What is the Formula for Futures Price?
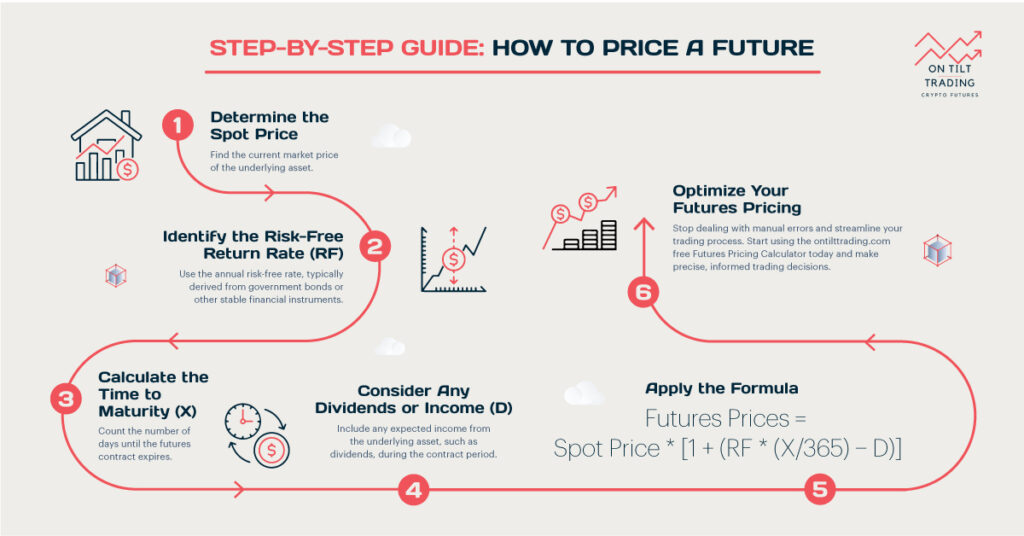
The pricing of futures contracts is a fundamental concept in financial markets. It is crucial in investment strategies, risk management, and market efficiency. At its core, the futures price is determined by a relationship between the current spot price of the underlying asset and various factors that influence its future value. The most commonly used formula for calculating futures prices is:
Futures Price = Spot Price * [1 + (RF * (X/365) – D)]
Where:
- Spot Price: The current market price of the underlying asset
- RF: Risk-free interest rate
- X: Number of days until the futures contract expires
- D: Dividend yield (for dividend-paying stocks) or storage costs (for commodities)
This formula, known as the cost-of-carry model, is based on the principle of arbitrage-free pricing. It assumes that the futures price should reflect the cost of holding the underlying asset until the contract’s expiration date.
Let’s break down each component of the formula:
Spot Price: This is the starting point for futures pricing. It represents the current market value of the underlying asset.
Risk-free interest rate (RF): This factor accounts for the time value of money. It represents the opportunity cost of holding the asset instead of investing in a risk-free alternative.
Time to expiration (X/365): This term adjusts the interest rate for the specific duration of the futures contract. It’s expressed as a fraction of a year.
Dividend yield or storage costs (D): For dividend-paying stocks, this term accounts for the dividends that would be earned by holding the actual shares. For commodities, it represents the storage costs associated with holding the physical asset.
The formula essentially states that the futures price should equal the spot price plus the cost of carrying the asset until the contract’s expiration, minus any benefits from holding the asset (such as dividends).
What are the Different Future Pricing Models?
While the cost-of-carry model is widely used, there are several other pricing models for futures contracts, each with its own assumptions and applications:
Cost-of-Carry Model
As described above, this is the most common model for pricing futures contracts. It’s particularly useful for financial futures and commodities that can be easily stored.

Expectancy Model
This model is based on market participants’ expectations of the future spot price. The futures price is seen as the market’s best estimate of what the spot price will be at the contract’s expiration. This model is often applied to commodities that are difficult to store or where supply and demand factors play a significant role.
Consider a grain like wheat, where storage is costly and difficult. If market participants expect the spot price of wheat to rise from $300 per ton to $350 per ton in six months due to anticipated poor harvests, the futures price would reflect this expectation, likely pricing around $350 per ton.
Convenience Yield Model
Convenience Yield Model: This model extends the cost-of-carry model by incorporating a “convenience yield” – the benefit of holding the physical asset rather than the futures contract. It’s particularly relevant for commodities where there might be temporary shortages or where holding the physical asset provides additional benefits.
Futures Price = Spot Price * [1 + (RF – CY) * (X/365)]
Where CY is the convenience yield.
Arbitrage Pricing Theory (APT): This model suggests that futures prices are influenced by multiple risk factors, not just the spot price and interest rates. It allows for a more complex analysis of pricing, considering various economic and market factors.
Black-Scholes Model Adaptation: While primarily used for options pricing, adaptations of the Black-Scholes model can be applied to futures pricing, particularly for more complex futures contracts or those with option-like features.
Stochastic Models: These advanced models incorporate random variables to account for the uncertainty in future spot prices. They’re particularly useful for commodities with high price volatility or when pricing long-term futures contracts.
Important Things to Keep in Mind While Analyzing Future Prices
Market Efficiency
Efficient markets should quickly incorporate all available information into futures prices. Any persistent deviations from theoretical prices may indicate market inefficiencies or additional risk factors not accounted for in basic models. Struggling with the complexities of futures pricing calculations? On Tilt
Trading’s free Futures Pricing Calculator simplifies the process, helping you accurately determine futures prices, manage risk, and make informed trading decisions effortlessly. Make smarter trading decisions today!
Contango and Backwardation
These terms describe the relationship between futures prices and expected future spot prices. In contango, futures prices are higher than expected future spot prices, while in backwardation, they’re lower. These conditions can significantly impact investment and hedging strategies.
Seasonality
For many commodities, seasonal patterns in supply and demand can have a substantial impact on futures prices. Understanding these patterns is crucial for accurate price analysis. For physical commodities, the cost of storage and the benefits of holding the physical asset (convenience yield) can significantly influence futures prices.
Interest Rate Environment
Changes in interest rates can have a direct impact on futures prices, particularly for financial futures and long-term contracts. Futures prices can be influenced by market sentiment and speculative trading, sometimes leading to prices that deviate from fundamental values.
Regulatory Changes
Changes in regulations, such as position limits or margin requirements, can impact futures prices and market dynamics.
For many futures contracts, particularly those based on commodities or currencies, global economic conditions play a crucial role in price determination. The liquidity of a futures contract can impact its pricing, with less liquid contracts potentially showing larger deviations from theoretical prices.
Some Essential Definitions Related to Pricing Futures Contracts
Spot Price
The spot price is the current market price for the immediate delivery of an asset. If the spot price of gold is $1,800 per ounce, you can buy or sell gold immediately at this price.
Forward Price
The forward price is the agreed-upon price in a forward contract for the future delivery of an asset. A company agrees to purchase copper at $4.50 per pound three months from now, irrespective of its market price at that time.
Marking to Market
Marking to market is the daily process of adjusting the margin account to reflect the current market value of futures positions. Silver futures contracts adjust daily to reflect changes in market prices, so your account balance changes accordingly.
Hedging
Hedging involves using futures contracts to reduce the risk of adverse price movements in an asset. A farmer sells wheat futures to lock in a price and protect against the risk of falling prices at harvest time.
Arbitrage
Arbitrage is the practice of simultaneously buying and selling related securities in different markets to profit from price discrepancies. A trader could buy gold in New York and sell it in London for a profit if gold’s price is lower in New York than in London.
Speculation
Speculation involves taking positions in futures contracts to profit from expected price movements, rather than for hedging purposes. A trader buys oil futures expecting the price to rise, intending to sell the contracts later at a higher price to make a profit.
Read More: What Happens If Future Price Is Less Than Spot Price?
Final Words
Understanding these concepts and the various pricing models is essential for anyone involved in futures trading or using futures for risk management. The complexity of futures pricing reflects the multitude of factors that can influence the value of an asset over time. You can avoid the risks using the right tools.